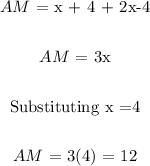Question

sorry it is blurry but this is what it say in ABC, centroid D is on the median AM AD=x+4 and DM=2x-4 Find AM

Answer 1

The measure of AM is 12

Here, we are to find the measure of AM

Mathematically, the centroid divides the median on which it lies into 2 parts

These parts are usually in the ratio 1 to 2

What this mean is that if we multiply the smaller side by 2, we get the larger side

That would proceed as follows;

`\begin{gathered} 2(2x-4)\text{ = x + 4} \\ 4x\text{ - 8 = x + 4} \\ \\ 4x-x\text{ = 8 + 4} \\ \\ 3x\text{ = 12} \\ \\ x\text{ = }(12)/(3) \\ \\ x\text{ = 4} \end{gathered}`

Now, the length of the median is the sum of the two parts

Mathematically, that would be;

`\begin{gathered} AM\text{ = x + 4 + 2x-4} \\ \\ AM\text{ = 3x} \\ \\ \text{Substituting x =4} \\ \\ AM\text{ = 3(4) = 12} \end{gathered}`