ANSWER

Step-by-step explanation
We want to find the equation of the line passing through (-3, 2) and parallel to:
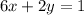
First, put the equation in slope intercept form:

A line that is parallel to another line has the same slope as that line.
Therefore, the slope of the required line is -3.
Now, use the point-slope method to find the equation of the line:
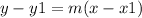
where (x1, y1) is the point the line passes through
Therefore, the equation of the line is:
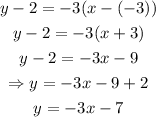
That is the equation of the line.