Question

If a:b=2:5 and b:c=3:4, find a:b:c

Answer 1

Rewrite the ratios in such a way that the number that corresponds to b is the same in both.

Since a:b = 2:5 and b:c = 3:4, then, the numbers that correspond to b are 5 and 3.

The least common multiple of 3 and 5 is 15. Multiply the first ratio by 3 and the second ratio by 5 in order to get two ratios with the same number for b:

`\begin{gathered} a:b=2:5 \\ =2\cdot3:5\cdot3 \\ =6:15 \\ \\ b:c=3:4 \\ =3\cdot5:4\cdot5 \\ =15:20 \end{gathered}`

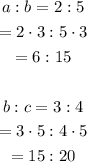
Since a:b = 6:15 and b:c = 15:20, then:

`a:b:c=6:15:20`

Therefore, the answer is: a:b:c = 6:15:20.