Question

What is the area of the triangle in the diagram?

Answer 1

A for plato users

Explanation:

Answer 2

The answer is the option A

`(1)/(2)(\sqrt{[(y2)^(2)+(x2)^(2)]*[(y1)^(2)+(x1)^(2)]})`

Explanation:

see the attached figure with letters to better understand the problem

we know that

The area of the triangle is equal to

`A=(1)/(2)bh`

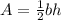
where

b is the base

h is the height

In this problem

`b=AB, h=AC`

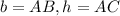
the formula to calculate the distance between two points is equal to

`d=\sqrt{(y2-y1)^(2)+(x2-x1)^(2)}`

Find the distance AB

`A(0,0), B(x2,y2)`

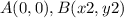
substitute

`dAB=\sqrt{(y2-0)^(2)+(x2-0)^(2)}`

`dAB=\sqrt{(y2)^(2)+(x2)^(2)}`

Find the distance AC

`A(0,0), C(x1,y1)`

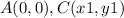
substitute

`dAC=\sqrt{(y1-0)^(2)+(x1-0)^(2)}`

`dAC=\sqrt{(y1)^(2)+(x1)^(2)}`

Find the area of the triangle

we have

`b=\sqrt{(y2)^(2)+(x2)^(2)}, h=\sqrt{(y1)^(2)+(x1)^(2)}`

substitute

`A=(1)/(2)(\sqrt{(y2)^(2)+(x2)^(2)})(\sqrt{(y1)^(2)+(x1)^(2)})`

`A=(1)/(2)(\sqrt{[(y2)^(2)+(x2)^(2)]*[(y1)^(2)+(x1)^(2)]})`