Question

You have been asked by the police department to find three locations the Acute Perps gang is likely to hit in the coming weeks. Because the gang sticks to a triangular pattern, the locations could be a translation, reflection, or rotation of the original triangle. For this step, identify and label three points on the coordinate plane that are a translation of the original triangle. Next, use the coordinates of your translation along with the distance formula to show that the two triangles are congruent by the SSS postulate. You must show all work with the distance formula.

Answer 1

The goal is to translate the triangle with the given vertices in any which you choose and then prove that the original triangle and the translated triangle are congruent by the side-side-side postulate (i.e., if 3 sides of a triangle are equal to 3 sides of another triangle (in length/distance) then the 2 triangles are congruent). For example, let's say you want to translate the given triangle 4 units to the right and 2 units down. Then you add 4 units to the x-coordinate of each vertex and subtract 2 units from the y-coordinate of each vertex to obtain the this translation. That is,    (3, 6)    --> (3+4, 6-2)=(7, 4)   (6, -3)   --> (6+4, -3-2)=(10, -5)   (-2, -3)  --> (-2+4, -3-2)=(2, -5) Now compute the length of each side of both triangles using the distance formula. If the lengths of the 3 sides of the original triangle are equal to the length of the 3 sides of the translated triangle, then they are congruent by the SSS postulate. 

Answer 2

Note:

Images with coordinates or original and translated triangles are attached.

Answer:

The coordinates for the given triangle are A(6,3), B(6,-3) and C(-2,-3), respectively.

Let us translate this triangle by -6 units along the x-axis and +3 units along the y-axis. This means that 6 will be subtracted from each of the abcissas (x-points) and 3 will be added to each ordinates (y-points). Hence, our translated triangle will have the following coordinates: A'(0,6), B'(0,0) and C'(-8,0), respectively.

Now to prove that both triangles are congruent, we use the SSS postulate, which states that:

"If three sides of one triangle are congruent to three sides of another triangle, then the triangles are congruent."

Distance between two point P1 and P2 will be:

`|P_1P_2|=√((x_2-x_1)^2+(y_2-y_1)^2)`

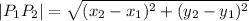
Now using distance formula to find sides of triangle ABC and triangle A'B'C'.

For Triangle ABC:

|AB| =
`√((6-6)^2+(-3-3)^2) =\;6\;units`

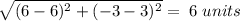
|AC| =
`√((-2-6)^2+(-3-3)^2) =\;10\;units`

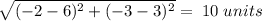
|BC| =
`√((-2-6)^2+[-3-(-3)]^2) =\;8\;units`

For Triangle A'B'C':

|A'B'| =
`√((0-0)^2+(0-6)^2) =\;6\;units`

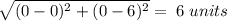
|A'C'| =
`√((-8-0)^2+(0-6)^2) =\;10\;units`

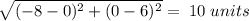
|B'C'| =
`√((-8-0)^2+(0-0)^2) =\;8\;units`

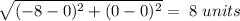
Hence, as the distances are equal, the two triangles are congruent by SSS postulate.