First, use the double angle formula for tangent:
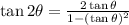
and then plug it in:
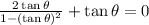
Multiply by
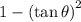
on both sides to get:


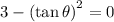

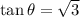
This means one solution is
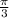
. To get the other solutions just add integer multiples of
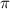
(because the period of tangent is pi so answers will repeat every pi).