Question

Remote Assignment 1-5 Score: 37/100 3/8 answered Progress saved Done Question 4 < > B0/13 pts 10 A BX bisects ZABC. If m ZABC = 9 x + 18 and mZCBX = 5 x + 7, find the value of mZABX. Submit Question

Answer 1

When a ray bisects an angle, it divides said angle in half. This means that the two new created angles will have the same measurement and when added they should be equal to the larger one. With this in mind we can find mABX as shown below:

`\begin{gathered} \measuredangle ABC=\measuredangle ABX+\measuredangle CBX \\ \measuredangle ABX=\measuredangle ABC-\measuredangle CBX \end{gathered}`

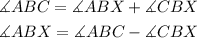
We were given the values for mABC and mCBX, so we use those in the expression above and solve for the value of mABX. We have:

`\begin{gathered} \measuredangle ABX=9x+18-(5x+7) \\ \measuredangle ABX=9x-5x+18-7 \\ \measuredangle ABX=4x+11 \end{gathered}`

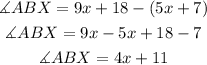
The value of mABX is "4x + 11".