Question

The sum of two integers is 42 and their product is 432.Write and solve an equation to find the two integers.

Answer 1

Let's call x and y as the required numbers.

Their sum is 42, thus:

x + y = 42

Their product is 432, thus:

xy = 432

From the first equation, solve for x:

x = 42 - y

Substitute in the second equation:

(42 - y) y = 432

Multiplying:

`42y-y^2=432`

Rearranging:

`-y^2+42y-432=0`

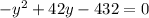
We use the quadratic formula to find the solutions to this equation. For a=-1, b=42, and c=-432:

`y=\frac{-42\pm\sqrt[]{42^2-4\cdot(-1)\cdot(-432)}}{2\cdot(-1)}`

Calculating:

`\begin{gathered} y=\frac{-42\pm\sqrt[]{1764-1728}}{-2} \\ y=\frac{-42\pm\sqrt[]{36}}{-2} \\ y=(-42\pm6)/(-2) \end{gathered}`

Separate the two roots:

y = 24 , y = 18

If we use the first value, then x = 42 - 24 = 18

If we use the second value, then x = 42 - 18 = 24

In either case, the two integers are 18 and 24