Question

10. It takes Michelle 25 minutes to descend 850 feet of the beginner slope at MountWashington, (5 points)a) Find her rate of descent (slope)b) If Michelle begins her descent at a height of 1200 feet, find a linear equation to describe herskiing motion

Answer 1

Part a

We have that it takes Michelle 25 minutes to descend 850 feet of the beginner slope. Then, we have that the rate of descent must be negative since she is descending constantly at the rate of the slope. Then, we have:

x1 = 0, y1 = 850ft

x2 = 25, y2 = 0

Then, the slope is:

`m=(y_2-y_1)/(x_2-x_1)\Rightarrow m=(0-850)/(25-0)(ft)/(\min)\Rightarrow m=-(850)/(25)(ft)/(\min )`

`m=-34(ft)/(\min )`

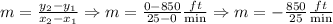
Then, her rate of descent (slope) is m = -34 ft/min.

Part b

If we have that Michelle begins her descent at a height of 1200 feet, and we have that her rate of descent is m = -34 ft/min, then we have that the linear equation that describes her skiing motion is (in slope-intercept form):

`y=mx+b`

• m = -34ft/min

,

• b is the y-intercept of the line. She starts her descent from 1200 feet.

Then we have:

`y=-34x+1200`

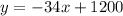
And we have that y is the descent from Mount Washington, in feet, and x is the time in minutes for the descent.

In summary, we have:

• Her rate of descent (slope) is -34 ft/min.

,

• The linear equation to describe her skiing motion is y = -34x + 1200.