Question

A population of beetles are growing according to a linear growth model. The initial population (week 0) isPo = 8, and the population after 6 weeks is P = 26.Find an explicit formula for the beetle population after n weeks.Pn=After how many weeks will the beetle population reach 74?weeks

Answer 1

We know that the population is modeled by a linear equation, this means that the model has the form:

`P(n)=mn+b`

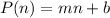
where m is the slope of the linear model and b is the intercept of the model (the initial population). Since the population in week zero is 8, this means that b=8 and we have:

`P(n)=mn+8`

To determine the value of m we use the fact that after 6 weeks (n=6) the population is 26, then:

`\begin{gathered} 6m+8=26 \\ 6m=18 \\ m=(18)/(6) \\ m=3 \end{gathered}`

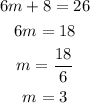
hence the slope is 6 (this means that each week there are three more beetles).

Therefore, the model of the population is given by:

`P(n)=3n+8`

To determine after how many weeks the population is 74 we equate our expression to 74 and solve for n:

`\begin{gathered} 3n+8=74 \\ 3n=66 \\ n=(66)/(3) \\ n=22 \end{gathered}`

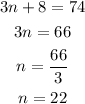
Therefore, it takes 22 weeks to have 74 beetles.