Question

Practice: An object is launched into the air with an initial velocity of 48ft/sec. from the topof a building 288 ft high. The height h(t) of the object after t seconds is given by h(t) =-16t2 + 48t + 288.

Answer 1

R)

Given the function of the height of the object, we can obtain its maximum by using its derivative, as shown below.

`\begin{gathered} h^(\prime)(t)=0\to\text{critical point} \\ h^(\prime)(t)=-32t+48 \\ \Rightarrow h^(\prime)(t)=0 \\ \Rightarrow-32t+48=0 \\ \Rightarrow t=(48)/(32)=(3)/(2) \end{gathered}`

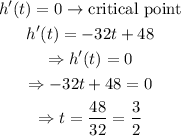
Then, the object will reach its maximum height at t=3/2, which is

`h((3)/(2))=-16((3)/(2))^2+48\cdot(3)/(2)+288=324`

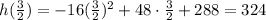
The time it takes for the object to reach its maximum height is 1.5 seconds.

The maximum height is 324 ft.