Given:
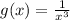
Required:
Sketch the graph for g(x) and find the total area between the curve g(x) and x-axis. Explain if necessary and provide a reason if the question cannot be solved.
Step-by-step explanation:
The given function is:
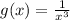
We can observe from the graph that there is no bounded area between the graph of the function and the x-axis.
To find the area between the x-axis and the curve the area should be bound with the x-axis.
So the area of the given function can not be calculated.
Final answer:
The question can not be solved because there is no bounded area between the graph of the function and the x-axis.