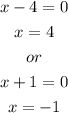Question

Once you are done factoring, how do you find the solutions (or x-intercepts) of the quadratic equation?

Answer 1

After factoring, to find the solutions of a quadratic equation, we have to apply the Zero Product rule by setting each of the factors to zero and solving for the variable.

The solutions of the given equation is x = 4 and x = -1

Step-by-step explanation:

Given the below quadratic equation;

`x^2-3x-4=0`

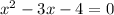
The first step to solving the above is to look for two factors of -4 whose sum will give -3. These numbers are -4 and 1. So let's go ahead and replace the middle term with these two factors as shown below;

`x^2-4x+x-4=0`

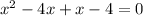
The 2nd step is to factorize;

`\begin{gathered} x(x-4)+(x-4)=0_{} \\ (x-4)(x+1)=0 \end{gathered}`

The 3rd step is to apply the Zero Product Rule, by setting each factor to zero and solving for x;

`\begin{gathered} x-4=0 \\ x=4 \\ or \\ x+1=0 \\ x=-1 \end{gathered}`