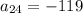Question

Write and equation to find the nth term of each sequence -4,-9, -14, -19 . Then find a24

Answer 1

We have here in this an arithmetic progression. We have that the common difference is d = -5. (If we add -5 to -4, we obtain the second term -9, and so on).

We have that the first term is -4.

Then, according to the equation for the arithmetic progressions, we have:

`a_n=a_1+(n-1)d`

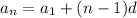
Then, the equation to find the nth term is the one above.

The equation for this case is:

`a_n=-4+(n-1)\cdot(-5)`

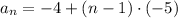
For example, we have in the sequence that the fourth term is -19. Then:

`a_4=-4+(4-1)\cdot(-5)\Rightarrow a_4=-4+(3)\cdot(-5)\Rightarrow a_4=-4-15\Rightarrow a_4=-19`

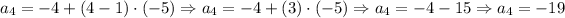
Therefore, to find the 24th element of the arithmetic progression, we have:

`a_(24)=-4+(24-1)\cdot(-5)\Rightarrow a_(24)=-4+(23)\cdot(-5)\Rightarrow a_(24)=-4-115_{}`

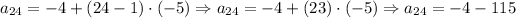
Then, the 24th element is:

`a_(24)=-119`