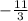Question

The sum of the root of 3x^2+11x-4=0

Answer 1

A product of several terms equals zero. When a product of two or more terms equals zero, then at least one of the terms must be zero. We shall now solve each term = 0 separately. In other words, we are going to solve as many equations as there are terms in the product. Any solution of term = 0 solves product = 0 as well.

Solve : 3x-1 = 0
Add 1 to both sides of the equation :
3x = 1
Divide both sides of the equation by 3:
x = 1/3 = 0.333

Solve : x+4 = 0 Subtract 4 from both sides of the equation : x = -4

x = -4 and x = 1/3 or 0.333

Answer 2

Option (a) is correct.

The sum of roots of given quadratic equation
`-(11)/(3)`

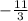
Explanation:

Given quadratic equation
`3x^2+11x+4=0`

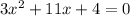
We have to find the sum of roots.

Consider the given quadratic equation
`3x^2+11x+4=0`

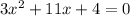
For a given standard quadratic equation
`ax^2+bx+c=0` , we have

Sum of roots is given by
`-(b)/(a)`

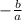
and product of roots is given by
`(c)/(a)`

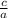
Thus, for the given quadratic equation
`3x^2+11x+4=0`

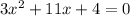
a = 3 , b = 11 , c = 4

So, the sum of roots is given by
`-(b)/(a)`

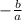
Substitute, we get,

Thus, The sum of roots of given quadratic equation
`-(11)/(3)`