Question

Find the measure of the numbered angles in rhombus DEFG

Answer 1

In a rhombus, the diagonals are perpendicular, which means all four triangles created are right triangles. Also, the diagonals bisects the angles, making all four triangles congruent.

Therefore we can write the following relations:

<1 = <2

<5 = <6

<1 + <6 = 90°

<5 + 27° = 90°

<4 = 27°.

First, let's calculate the angle <5:

`\begin{gathered} <5+27°=90°\\ \\ <5=90-27\\ \\ <5=63° \end{gathered}`

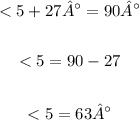
From this value, we also have that <6 = 63°. Then, we can find that <1 = 27°, and then <2 = 27°.

<3 is an angle created in the intersection point of the diagonals, therefore <3 = 90°.

So the answer is:

<1 = 27°, <2 = 27°, <3 = 90°, <4 = 27°, <5 = 63°, <6 = 63°.