Question

Ming has some money to invest in one of two different accounts for 3 years.Account 1 requires an investment of $700 and earns 6.2% interest, compounded quarterly.Account 2 requires an investment of $800 and earns 5.1% interest, compounded monthly.Assuming that Ming has enough money to make either investment, which account has a higher return?Select the answer that is completely correct.Account 1 because it earns about $10 more than Account 2Account 1 because it earns about $8 more than Account 2Account 2 because it earns about $92 more than Account 1Account 2 because it earns about $90 more than Account 1

Answer 1

Account 1 because it earns about $10 more than Account 2

Step-by-step explanation

to solve this we can find the amounts and then compare them, so

Step 1

Account 1 requires an investment of $700 and earns 6.2% interest, compounded quarterly

so

to find the future value we need apply the formula

`\begin{gathered} A=P(1+(r)/(n))^(nt) \\ where \\ A\text{ is the amount ( future value)} \\ P\text{ =Principal ( initial amount)} \\ r=\text{interest rate} \\ n=\\u mber\text{ of compounded times in one`

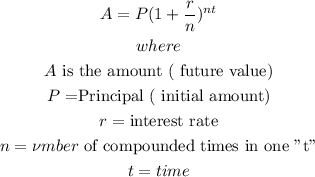
then, let

`\begin{gathered} A=\text{unknown} \\ P\text{ =7}00 \\ r=\text{6}.2\text{ \% = 0.062} \\ n=4(\text{ it is quartelyr)} \\ t=3\text{ years} \end{gathered}`

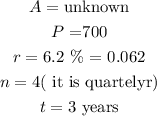
replace and evaluate

`\begin{gathered} A=P(1+(r)/(n))^(nt) \\ A=700(1+(0.062)/(4))^(4\cdot3) \\ A=700(1.0155)^(12) \\ A=841.89 \end{gathered}`

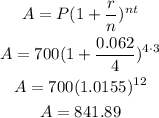
it means that for account 1, the earning is

`\begin{gathered} \text{Earning}=\text{ actual-initial } \\ \text{Earning}=841.89-700=141.89 \end{gathered}`

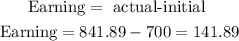
account 1: 141.89

Step 2

now, for account 2

Account 2 requires an investment of $800 and earns 5.1% interest, compounded monthly.

Let

`\begin{gathered} A=\text{unknown} \\ P\text{ =8}00 \\ r=\text{5}.1\text{ \% = 0.05}1 \\ n=12(monthly\text{)} \\ t=3\text{ years} \end{gathered}`

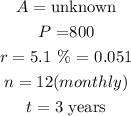
replace and evaluate

`\begin{gathered} A=P(1+(r)/(n))^(nt) \\ A=800(1+(0.051)/(12))^(12\cdot3) \\ A=800(1.00425)^(36) \\ A=800(1.164) \\ A=931.957 \end{gathered}`

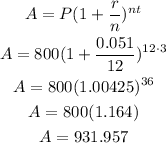
so, the earnings in option2 would be:

`\begin{gathered} \text{Earning}=\text{ actual-initial } \\ \text{Earning}=931.957-800=131.96 \end{gathered}`

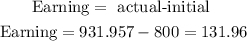
Step 3

finally, let's compare the earnings

`\begin{gathered} \text{Option 1 :141.89} \\ \text{Option 2: 131.96} \\ \text{Option 1 -option 2=141.89-131.96}\approx10 \\ so,\text{ difference is about 10} \\ \text{account 1 is 10 more than account 2} \end{gathered}`

therefore, the answer is

Account 1 because it earns about $10 more than Account 2

I hope this helps you