Question

The grades on the last math exam had a mean of 72%. Assume the population of grades on math exams is known to be distributed normally, with a standard deviation of 5%. Approximately what percent of students earn a score between 72% and 87%?

Answer 1

Approximately 50%.

Explanation:

We have been given that the grades on the last math exam are normally distributed and had a mean of 72% and a standard deviation of 5%.

To find the percent of students, who will earn a score between 72% and 87% we will use z-score formula.

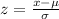`z=(x-\mu)/(\sigma)`, where,

`z=\text{z-score}`,

`x=\text{Random sample score}`,

`\mu=\text{Mean}`,

`\sigma=\text{Standard deviation}`.

Let us find z-score corresponding to sample score of 72%.

`z=(72\%-72\%)/(5\%)`

`z=(0\%)/(5\%)`

`z=0`

Let us find z-score for sample score 87%.

`z=(87\%-72\%)/(5\%)`

`z=(15\%)/(5\%)`

`z=3`

Let us find the probability of z-score between 0 and 3.

Since we now that probability between two z-scores can be found by subtracting the probability of smaller area from bigger area.

`P(a<z<b)=P(z<b)-P(z<a)`

Upon substituting our given values in above formula we will get,

`P(0<z<3)=P(z<3)-P(z<0)`

Using normal distribution table we will get,

`P(0<z<3)=0.99865-0.50000`

`P(0<z<3)=0.49865`

Let us convert our given probability to percentage by multiplying 0.49865 by 100.

`0.49865*100=49.865\%\approx 50\%`

Therefore, approximately 50% of students will earn a score between 72% and 87%.

Answer 2

49.865%
round to the nearest tenth of a percent: 49.9%
round to the nearest integer: 50%