Question

Solve, graph and write the solution in interval notation: |6x−5|<7

Answer 1

The given inequality is,

`|6x-5|<7`

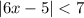
According to absolute rule,

`|y|0 then y>-a and y}Applying absolute rule to the given inequality, [tex]6x-5>-7\text{ and }6x-5<7`

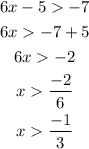
Solving 6x-5>-7,

`\begin{gathered} 6x-5>-7 \\ 6x>-7+5 \\ 6x>-2 \\ x>(-2)/(6) \\ x>(-1)/(3) \end{gathered}`

Solving 6x-5<7,

`\begin{gathered} 6x-5<7 \\ 6x<7+5 \\ 6x<12 \\ x<(12)/(6) \\ x<2 \end{gathered}`

So, the solution is x>-1/3 and x<2.

The solution in interval notation is (-1/3, 2).

Now, the graph of the inequality is,

(Hollow dot in the graph indicates open interval).