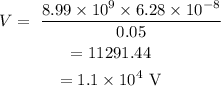Question

A 5 cm radius conducting sphere has a charge density of 2.0x10-6 C/m2 on its surface. Find the electric potential of the sphere.7.2x10^6 V1.1x10^4V3.6x10^5 V2.3x10^5 V2.2x10^4 V

Answer 1

Given that the radius of the conducting sphere is r = 5 cm = 0.05 m

The charge density on the surface of the sphere is

`\sigma\text{ = 2}*(10^(-6)C)/(m^2)`

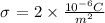
We have to find the electric potential.

To calculate electric potential, first, we need to calculate charge.

The charge will be

`\begin{gathered} \sigma=(Q)/(4\pi r^2) \\ Q=4\pi r^2*\sigma \\ =\text{ 4}*3.14*(0.05)^2*2*10^(-6) \\ =\text{ 6.28}*10^(-8)\text{ C} \end{gathered}`

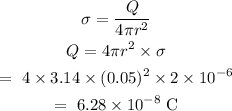
The electric potential is given by the formula

`V=\text{ k}(Q)/(r)`

Here K is Coulomb's constant whose value is

`K=\text{ 8.99 }*(10^9Nm^2)/(C^2)`

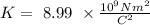
Substituting the values, the electric potential will be

`\begin{gathered} V=\text{ }(8.99*10^9*6.28*10^(-8))/(0.05) \\ =11291.44 \\ =1.1*10^4\text{ V} \end{gathered}`