Question

If y varies inversely as x and y=2 when x=8, find x when y =14

Answer 1

When we have the statement " y varies inversely as x ". We have the next equation:

`y=(k)/(x)`

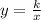
Where k represents the constant between them.

Replace using the given values:

y=2

x=8

`2=(k)/(8)`

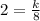
Now, solve for k:

Multiply both sides by 8

`\begin{gathered} 8\cdot2=8\cdot(k)/(8) \\ 16=k \end{gathered}`

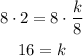
Hence, the constant value k is equal to 16.

Let's find x where y=14 and k =16.

Use the same equation to find x and replace it with the given values:

`\begin{gathered} 14=(16)/(x) \\ \text{Multiply both sides by x} \\ x\cdot14=x\cdot(16)/(x) \\ \text{Simplify} \\ 14x=16 \\ \text{Now, divide both sides by 14} \end{gathered}`
`\begin{gathered} (14)/(14)x=(16)/(14) \\ x=(8)/(7) \end{gathered}`

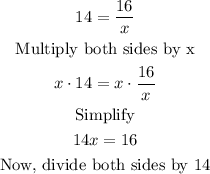
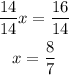
Hence, when y=14, the x value will be 8/7