Answer:
(a)C=25+0.25n
(b)Graph A
(c)$37.50
(d)40 minutes
Step-by-step explanation:
Part A
Monthly Fee = $25
Cost per minute = 25 cents = $0.25
Therefore, an equation for the total monthly cost C when n minutes are used is:
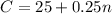
Part B
The equation C=25+0.25n has the following properties:
• Rate of change = 0.25
,
• y-intercept = 25
From the given graphs, the one that satisfies these properties is Graph A shown below:
Part C
If 50 min of long-distance calls is made, the estimated monthly cost is circled in red above.
• Trace it to the y-axis
,
• It is halfway between 35 and 40.
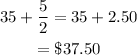
The estimated monthly cost for 50 mins of long-distance calls is $37.50.
Part D
If the total monthly bill is $35
From the graph, trace the point circled in green to the x-axis.
The number of mins used for long-distance calls is 40 minutes.