Question

The lens equation is 1/f=1/p+1/q , where f is the focal length of the lens, p is the distance of the object from the lens, and q is the distance of the image from the lens. The formula to find q is:

a. q=pf/p-f
b. q=p-f/pf
c. q=pf/p+f

Answer 1

1/f=1/p + 1/q
least comom multiple=pfq
(pq)/(pfq)=(fq)/(pfq)+(pf)/(pfq)
Because all denominators are the same, we can eliminate the denominators.
pq=fq+pf
pq-fq=pf
q(p-f)=pf
q=pf / (p-f)

Answer: a. q=pf / (p-f)

Answer 2

`q=(pf)/(p-f)`

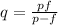
Explanation:

The lens equation is
`(1)/(f)=(1)/(p)+(1)/(q)`.

To solve this formula for
`q`, we need to multiply each term by
`fpq`.

`(fpq)(1)/(f)=(fpq)(1)/(p)+(fpq)(1)/(q)`.

We cancel out the common factors to get;

`pq=fq+fp`.

We group the terms in q on one side of the equation;

`pq-fq=fp`.

Factor q.

`q(p-f)=fp`.

Divide both sides by
`(p-f)`

`q=(pf)/(p-f)`

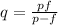
The correct answer is A