Question

You volunteer to help drive children at a charity event to the zoo, but you can fit only 6 of the 17 children present in your van How many different groups of 6 childrencan you drive?

Answer 1

Since we need to choose groups of 6 from 17 options, we call this "17 choose 6". This is the same as the nCr formula for "n choose r", so we need to use:

`\begin{gathered} C(n,r)=(n!)/(r!(n-r)!) \\ C(17,6)=(17!)/(6!(17-6)!)=(17!)/(6!\cdot11!) \end{gathered}`

Since factorial is the multiplication of the factors from the given one to 1, 17! divided by 11! cancels out all the factors from 11 to 1 of the 17!, so we are left with:

`C(17,6)=(17!)/(6!(17-6)!)=(17!)/(6!\cdot11!)=(17\cdot16\cdot15\cdot14\cdot13\cdot12)/(6\cdot5\cdot4\cdot3\cdot2\cdot1)`

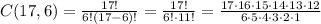
Now, we can cancel some factors out before evaluating everthing:

`C(17,6)=(17!)/(6!(17-6)!)=(17!)/(6!\cdot11!)=(17\cdot16\cdot15\cdot14\cdot13\cdot12)/(6\cdot5\cdot4\cdot3\cdot2\cdot1)=(17\cdot4\cdot1\cdot14\cdot13\cdot1)/(1\cdot1\cdot1\cdot1\cdot1\cdot1)=17\cdot4\cdot14\cdot13=12376`

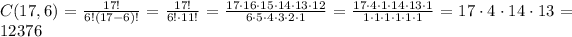
So, the number of combinations is 12376.