Question

What type of transformation takes the graph of f(x)=|x|f(x)=|x| to the graph of g(x)=2.5|x|g(x)=2.5|x|? vertical shift down of 2.5 vertical stretch by a factor of 2.5 vertical compression by a factor of 2.5 vertical shift up of 2.5

Answer 1

When you apply to the graph of the function
`y=f(x)` following transformations

• vertical shift by a factor a;
• vertical compression by a factor a;
• vertical shift up a units;
• vertical shift down a units,

then you will get the function

• 
  `y=a\cdot f(x),` where
  `|a|>1;`
• 
  `y=a\cdot f(x),` where
  `0<|a|<1;`
• 
  `y=f(x)+a;`
• 
  `y=f(x)-a.`

In your case, the graph of the function
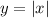`y=|x|` was vertically shifted by a factor of 2.5 to form the graph of the function
`y=2.5|x|.`

Answer: correct choice is B

Answer 2

vertical stretch by a factor of 2.5