Assume that the number of child tickets on Saturday is x
Since the number of adult tickets is twice the number of the child tickets, then
The number of adult tickets is 2x
Since the child admission is $5.30
Since the adult admission is $9.30
Since the total amount of admission is $860.40, then
Multiply x by 5.30 and 2x by 9.30, then add the products and equate the sum by 860.40
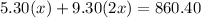
Simplify the left side
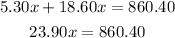
Divide both sides by 23.90
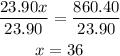
The number of child tickets sold on Saturday was 36