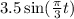Step-by-step explanation
So we have a weight oscillating due to the action of a spring. We are told that its period (i.e. the time it takes it to complete a cycle) is 6 seconds and the diference between its two extreme positions is 7 in. This kind of movement can be modeled with a sinusoidal equation i.e. an expression with a sine. Let's have a look at the following function:
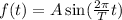
Where T is the period of the function and A is known as the amplitude. As we saw before, the period of our function must be equal to 6 because it takes the weight 6 seconds to complete a full cycle. Then we have:
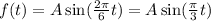
In order to find A let's take into account the behaviour of the sine. The maximum value of the sine is 1 and the minimum is -1. Then the extreme values of the function are A and -A. This are also the highest and lowest position of the weight. We are told that their difference must be equal to 7 in so we have the following equation for A:
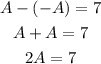
We divide both sides by 2 and we obtain A:
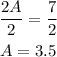
Therefore we get:
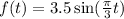
Answer
Then the answer is: