Question

3. Given a circle with a radius of 4 inches and a central angle of 170°, find the arclength and the sector area.

Answer 1

Use the following formula for the sector area:

`A=(\theta)/(360)\cdot\pi r^2`

where,

θ = 170

π = 3.14

r: radius = 4 in

Replace the previous values of the parameters into the formula for A and simplify:

`A=(170)/(360)(3.15)(4in)^2=23.8in^2`

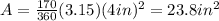
Hence, the sector area is 23.8 in^2

To find the measure of the arclength, use the following formula:

`s=2\pi r\cdot(\theta)/(360)=2(3.14)(4in)\cdot(170)/(360)\approx11.86in`

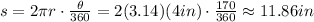
Hence, the arclength is approximately 11.86 in