If a line is written in the form:
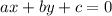
And the coordinates of a point are:
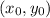
Then, the distance between the line and those points, is:
![d=\frac{\lvert ax_0+by_0+c\rvert}{\sqrt[]{a^2+b^2}}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/jg759llqgi2xvj7ffqi7.png)
a)
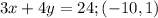
First, rewrite the equation in general form setting it equal to 0 by substracting 24 from each side:
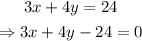
Comparing this equation to the general form of a line, we know that:
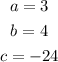
Substitute those values and the coordinates (-10,1) into the line-point distance formula:
![\begin{gathered} d=\frac{\lvert3(-10)+4(1)-24\rvert}{\sqrt[]{3^2+4^2}} \\ =\frac{\lvert-30+4-24\rvert}{\sqrt[]{9+16}} \\ =\frac{\lvert-50\rvert}{\sqrt[]{25}} \\ =(50)/(5) \\ =10 \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/7dgmmlk3widr9j965jhk.png)
Then, the distance between the given line and the given point in part a), is 10.
Apply a similar procedure to find all other distances.