Question

A certain radioactive isotope has leaked into a small stream. Three hundred days after the leak, 11% ofthe original amount of the substance remained. Determine the half-life of this radioactive isotope.

Answer 1

By the law of radioactive decay, the amount of a certain radioactive isotope as a function of time is given by

`N(t)=N_0e^(-\lambda t)`

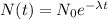
Where N_0 represents the initial amount of the substance, N represents the amount of the substance given a time t, and lambda is the decay constant.

This isotope that has leaked, started with an amount N_0, and after 300 days remained only 11% of it, which means that

`N(300)=0.11N_0`

If we evaluate 300 in our function, we're going to have

`N(300)=N_0e^(-300\lambda)`

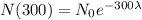
If we compare those two results, we have

`0.11N_0=N_0e^(-300\lambda)`

Dividing both sides by the initial amount.

`0.11=e^(-300\lambda)`

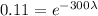
Solving for lambda, we have

`\begin{gathered} 0.11=e^(-300\lambda) \\ \ln (0.11)=-300\lambda \\ \lambda=-(300)/(\ln(0.11)) \\ \lambda=135.914198185\ldots \end{gathered}`

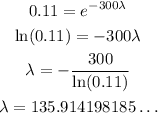
The decay rate and the half life of a isotope are related by the following formula

`\lambda=(\ln2)/(t_(1/2))`

Where t_(1/2) is the half life of the isotope.

Using our value for lambda, we have

`\begin{gathered} 135.914198185\ldots=(\ln2)/(t_(1/2)) \\ t_(1/2)=\ln 2\cdot135.914198185\ldots \\ t_(1/2)=94.20854327\approx94 \end{gathered}`

The half life of this substance is approximately 94 days.