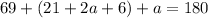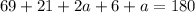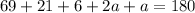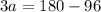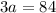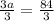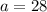Question

I need help finding the value of a. I can not figure out how to do this problem

Answer 1

Step-by-step explanation:

Given;

We are given a triangle divided into two right triangles as indicated.

We shall begin by sketching both triangles as shown below;

The triangle has now been decomposed into two right triangles.

Required;

We are required to calculate the value of a.

Step-by-step solution;

Take note that for any right angled triangle, the sum of the other two acute angles is always equal to 90 degrees. This is because, the sum of angles in a triangle is always equal to 180 degrees. If one angle is 90 degrees, then the other two angles will be equal to 90 degrees.

Therefore, we can set up the following equation;

`\begin{gathered} In\text{ }\Delta ADC: \\ \angle A+\angle D+\angle C=180\degree \end{gathered}`
`(2a+6)+a+90=180`
`2a+6+a=180-90`
`3a+6=90`
`3a=90-6`
`3a=84`

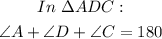
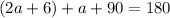
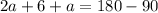
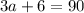
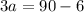
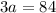
Divide both sides by 3;

`(3a)/(3)=(84)/(3)`
`a=28`

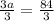
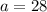
ANSWER:

The correct answer is option A

`a=28\degree`

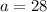
METHOD 2:

Having determined that the angle at the bottom left is 69 degrees, that is;

We can now take the entire triangle (without having to decompose it), and the three angles would be;

`\begin{gathered} \angle A=69\degree \\ \angle B=21+(2a+6) \\ \angle C=a \end{gathered}`

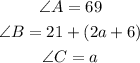
Note that the sum of all three angles equals 180 (angles in a triangle).

We now have the following equation;

`69+(21+2a+6)+a=180`
`69+21+2a+6+a=180`
`69+21+6+2a+a=180`
`96+3a=180`
`3a=180-96`
`3a=84`
`(3a)/(3)=(84)/(3)`
`a=28`