Question

A game has a rectangular board with an area of 44in2. There is a square hole near the top of the game board in which you must not toss in a bean bag. The square has side lengths of 3in. What is the probability of tossing the bag through the hole?

Answer 1

Answer: The required probability is 20.45%.

Step-by-step explanation: Given that a game has a rectangular board with an area of 44 in² and a square hole near the top of the game board in which we must not toss in a bean bag. The square has side lengths of 3 in.

We are to calculate the probability of tossing the bag through the hole.

The area of the rectangular board, A = 44 in²

and

the area of the square hole, a = 3 × 3 = 9 in².

Therefore, the probability of tossing the bag through the hole is given by

`P=(a)/(A)=(9)/(44)=0.2045=20.45\%.`

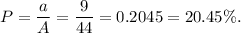
Thus, the required probability is 20.45%.

Answer 2

The area of the hole would be 9 in^2 if it is considered that every space withing the board has equal possibilities then

9/44 = 0.204 = 20.4%