Question

#9 label A and B parts and solve each separately to come to the result in different ways

Answer 1

9 (a) Using product rule

`f(x)=(x-1)(3x+4)`
`\begin{gathered} u=x-1 \\ (du)/(dx)=1 \end{gathered}`
`\begin{gathered} v=3x+4 \\ (dv)/(dx)=3 \end{gathered}`

The product rule formula is quoted below

`(dy)/(dx)=u(dv)/(dx)+v(du)/(dx)`
`\begin{gathered} f^(\prime)(x)=(dy)/(dx)=(x-1)*3+(3x+4)*1 \\ \\ f^(\prime)(x)=3(x-1)+3x+4 \\ f^(\prime)(x)=3x-3+3x+4 \\ f^(\prime)(x)=6x+1 \end{gathered}`

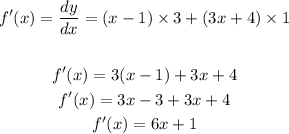
9(b) Verifying the resuts of 9(a) by bexpanding the product first and subsequently differentiating

`\begin{gathered} f(x)=(x-1)(3x+4) \\ f(x)=3x^2+x-4 \\ \\ f^(\prime)(x)=(dy)/(dx)=6x+1 \end{gathered}`

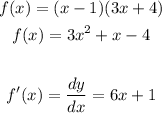
In conclusion , we observe that the results of the derivatives are the same irrespective of the approach used as shown in 9(a) and 9(b) above