Since the terms in the polynomial 27y³ - 8 are both perfect cubes, then we can use the Difference of Cubes Formula which is:
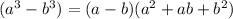
Since the terms in our polynomial are perfect cubes, we can rewrite the equation into:
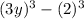
in which a = 3y and b = 2. Therefore, the factors of the polynomial are:
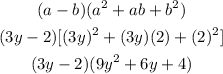
The factors are (3y - 2) and (9y² + 6y + 4).