Visualizing the given, we have
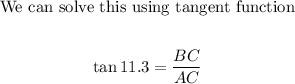
Find BC
Convert Tamara's height into feet first
62 inches → 31/6 feet
BC = 15 feet - 31/6 feet
BC = 59/6 feet
Now that we have BC, we can solve for AC (which is the distance from the flagpole to where Tamara is standing.

Rounding off to the nearest 2 decimal place.
The flagpole is 49.21 feet to where Tamara is standing.