a)
Since the value of the car decreases by 6% each year, then, the car preserves 94% of the value it had the previous year.
Then, after one year, the new price can be found by multiplying the previous price times a factor of 0.94.
After t years, this process occurs t times, then, the final price is the initial price multiplied by (0.94)^t.
Then, a general decay model for the value of the car in t years is:

b)
To find the value for the car that this model predicts 20 years in the future, replace t=20:

Therefore, the value of the car in 20 years will be $49,608.17 according to the model.
c)
Half of the original value is the same as $85,500.
To find the time that it will take for the car to reach that value, replace y=85,500 and solve for t:
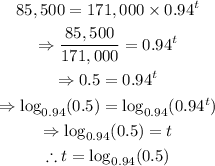
Use a calculator to find a decimal expression for t:
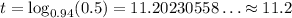
Therefore, the value of the Mercedes will reach half its original value after 11.2 years.