Question

THIS IS HIGH SCHOOL CALCULUS PROBLEM! MUST SHOW ALL THE JUSTIFICATION!!!

Answer 1

Given the graph of the function "f", you need to find the approximate output value:

`\begin{gathered} f(3.9) \\ f(4.04) \end{gathered}`

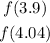
Notice that both values are closed to:

`x=4`

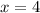
Therefore, you can use this formula:

`f(x+\Delta x)=f(x)+f^(\prime)(x)\Delta x`

In this case, you can approximate that:

`f(x)=x+c`

Where "c" is a constant.

Its derivative is:

`f^(\prime)(x)=1`
`f^(\prime)(x)=1`

(a) In order to find:

`f(3.9)`

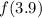
You need to use:

`\Delta x=4-3.9=0.1`

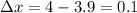
Then, using the formula, you get:

`f(3.9)\approx4+(1)(0.1)\approx4.1`

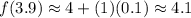
(b) And for the other value:

`\Delta x=4-4.04=-0.04`

Then:

`f(4.04)\approx4+(1)(-0.04)\approx3.96`

Hence, the answers are:

(a)

`f(3.9)\approx4.1`

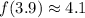
(b)

`f(4.04)\approx3.96`