Question

BD bisects m∠ABC if m∠ABD = (6x+4) and m∠DBC = (8x-4)

Answer 1

As you can see in the picture, m∠ABD = m∠DBC
So,


`m \angle ABD = m\angle DBC\\\\6x+4=8x-4\\\\4+4=8x-6x\\\\8=2x\\\\ (8)/(2)=x\\\\4=x\\\\ \therefore\boxed{x=4}`

Answer 2

x=4

Explanation:

BD is the line that bisects m∠ABC

When a line bisects and angle it form two angles and they are equal.

m∠ABD = m∠DBC

Plug in the measures of angles and solve for x

`6x+4= 8x-4`

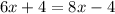
Subtract 6x from both sides

`4=2x-4`

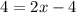
Add 4 on both sides

`8=2x`

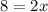
Divide both sides by 2

x= 4

The value of x=4