Answer:
1 of 5) D, 2 of 5) B, 3 of 5) D, 4 of 5) A, 5 of 5) D
Step-by-step explanation:
1 of 5) The composite area is the sum of the areas of two rectangles. That is:
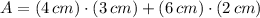
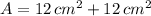

The area of the shaded region is 24 square centimeters. (Answer: D)
2 of 5) The composite area is the sum of the areas of the rectangle and the triangle. That is:
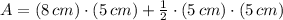
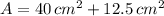
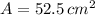
The area of the shaded region is 52.5 square centimeters. (Answer: B)
3 of 5) The composite area is the sum of the areas of the rectangle and the triangle. That is:
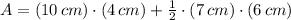
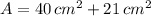

The area of the shaded region is 61 square centimeters. (Answer: D)
4 of 5) The composite area is the sum of the areas of four triangles and two rectangles. That is:
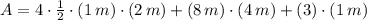

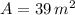
The area of the shaded region is 39 square meters. (Answers: A)
5 of 5) The composite area is the sum of the areas of the three semicircles and the square. That is:
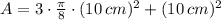

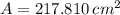
The area of the shaded region is 217.810 square centimeters. (Answer: D)