Question

Find the market equilibrium of the following:Supply: p=q2 + 14q – 529Demand: p =311-5qMarket equilibrium point:

Answer 1

The market equilibrium point is x + 21y

Explanation:

Given information

`\begin{gathered} \text{supply; p = q}^2\text{ + 14q - 529} \\ \text{Demand; p = 311- 5q} \end{gathered}`

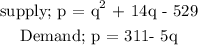
At the market equilibrium, quantity demand is equal to quantity supply

The next step is to equate the two equations together

`311-5q=q^2\text{ + 14q - 529}`
`\begin{gathered} 311-5q=q^2\text{ + 14q - 529} \\ q^2\text{ + 14q - 529 - 311 + 5q = 0} \\ \text{collect the like terms} \\ q^2\text{ + 14q + 5q - 840 = 0} \\ q^2\text{ + 19q - 840 = 0} \end{gathered}`

The next process is to solve for q using the general quadratic formula

`\begin{gathered} x\text{ =}\frac{-b\text{ }\pm\sqrt[]{b^2\text{ - 4ac}}}{2a} \\ \end{gathered}`

Where

a = 1, b = 19 and c = -840

`\begin{gathered} q\text{ =}\frac{-(19)\pm\sqrt[]{19^2-\text{ 4(1 }*-840)}}{2\text{ }*1} \\ q\text{ = }\frac{-19\text{ }\pm\sqrt[]{361\text{ - 4(-840)}}}{2} \\ q\text{ = }\frac{-19\text{ }\pm\sqrt[]{361\text{ + 3360}}}{2} \\ q\text{ = }\frac{-19\pm\sqrt[]{3721}}{2} \\ q\text{ = }(-19\pm61)/(2) \\ q\text{ = }\frac{-19\text{ + 61}}{2}\text{ OR }\frac{-19\text{ - 61}}{2} \\ q\text{ = }(42)/(2)\text{ or }(-80)/(2) \\ q\text{ = 21or -40} \\ q\text{ = 21} \end{gathered}`

From the above calculations, you will see that the value of q is 21 or - 40

Therefore, the market equilibrium price is 21

Recall that, the general function for the market equilibrium point is

Q(s) = x + yP

where p = price

Hence, the market equilibrium point is q(s) = x + 21y