Question

Triangles ADC and BCD are shown below. A B Which additional fact is needed to prove A ADC ABCD by the hypotenuse leg theorem? (G.6) (1 point) O A. AD BC O B. ZA ZB OC. ACBD OD. MZA=mZB = 45

Answer 1

Triangles ADC and BCD are both right angled triangles. The angles D and C are both 90 degrees (right angles), and line DC is common to both triangles.

Since line DC is common to bot triangles, then the following ratio applies;

`\begin{gathered} (AC)/(DC)=(BD)/(DC) \\ Also, \\ (AD)/(DC)=(BC)/(DC) \\ \text{Therefore, having the same ratio for both hypotenuse, } \\ \text{The lines} \\ AC\cong BD \end{gathered}`

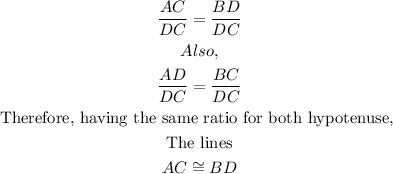
The ratio for the hypotenuse divided by the base is equal for both triangles, hence they both are congruent.