Given:
Number of ways, n = 21
Let's use counting principle to determine the number of elements in the sample space.
Given that we have a true-false question here, there are two possible outcomes to a true-false question.
Here, we are given 21 questions.
The counting principle states that for a scenario, the sequence for events which are independent is:
a1, a2, a3, a4, ..., an
For the number of ways the events can occur, wehave:
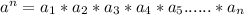
Given that a true-false question has out possible outcomes and we have 21 questions, the possible ways will be:

Therefore, there are 2097152 ways to