Question

The function y=f(x) is graphed below. Plot a line segment connecting the points on f where x=3x=3 and x=8. Use the line segment to determine the average rate of change of the function f(x) on the interval 3≤x≤8

Answer 1

Given:

The interval is,

`3\leq x\leq8`

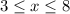
To find:

The average rate of change of the function

Step-by-step explanation:

Fromthe graph,

When x = 3,

`f(3)=-15`

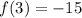
When x = 8,

`f(8)=5`

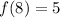
The graph is,

The formula for the average rate of the function is,

`Average\text{ }rate=(\Delta y)/(\Delta x)`
`\begin{gathered} \Delta y=f\mleft(b\mright)-f\mleft(a\mright) \\ =f(8)-f(3) \\ =5-(-15) \\ \Delta y=20 \end{gathered}`
`\begin{gathered} \Delta x=b-a \\ =8-3 \\ =5 \end{gathered}`

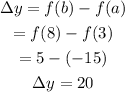
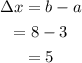
On substitution we get,

`\begin{gathered} Average\text{ }rate=(20)/(5) \\ =4 \end{gathered}`

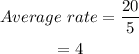
Final answer:

The average rate of change of the function is 4.