Question

What is the ratio of the sides of triangle ABYNEED HELP ASAP

Answer 1

GIVEN:

We are given two right triangles as indicated and these are;

`\begin{gathered} \Delta ABY\text{ } \\ \\ \Delta YXZ \end{gathered}`

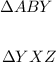
Required;

To find the ratio of the sides of the triangle ABY.

Step-by-step solution;

We shall take into consideration the values of the special angles as shown in the right triangles and these are as follows;

`\begin{gathered} Angle\text{ }30\degree: \\ \\ sin30\degree=(1)/(2) \\ \\ cos30\degree=(√(3))/(2) \\ \\ tan30\degree=(1)/(√(3)) \end{gathered}`
`\begin{gathered} Angle\text{ }60\degree: \\ \\ sin60\degree=(√(3))/(2) \\ \\ cos60\degree=(1)/(2) \\ \\ tan60\degree=√(3) \end{gathered}`
`\begin{gathered} Angle\text{ }45\degree: \\ \\ sin45\degree=(√(2))/(2) \\ \\ cos45\degree=(√(2))/(2) \\ \\ tan45\degree=1 \end{gathered}`

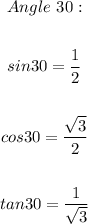
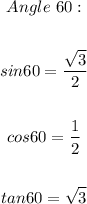
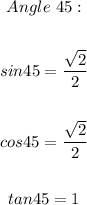
Observe carefully that the line AB is also the OPPOSITE of angle 30 degrees. This means line AB can also take on the value 1

That is,

`AB=1`

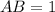
Then the line BY is the hypotenuse of triangle ABY and its length is 2.

That is;

`BY=2`

Line YA is the ADJACENT of triangle ABY and its length is √2.

That is;

`YA=√(2)`

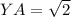
These can be better illustrated as follows;

Therefore, from the side lengths derived as shown above, the ratio of the sides of triangle ABY is as follows;

`1:2:√(2)`

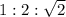
ANSWER:

The fourth option is the correct answer.