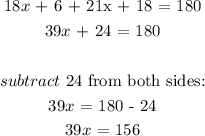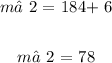Question

In the figure below, if ∠2=(18+6)°m∠2=(18x+6)° and ∠3=(21+18)°m∠3=(21x+18)°then ∠2=m∠2= ____ .

Answer 1

Answer:

m∠2 = 78°

Step-by-step explanation:

Given:

m∠2 = (18x + 6)°

m∠3 = (21x + 18)°

To find:

m∠2

From the diagram, m∠2 and m∠3 are the same side interior angles. Same-side interior angles sum up to 180 degrees as they are supplementary angles. As a result,

m∠2 + m∠3 = 180°

(18x + 6)° + (21x + 18)° = 180°

`\begin{gathered} 18x\text{ + 6 + 21x + 18 = 180} \\ 39x\text{ + 24 = 180} \\ \\ subtract\text{ 24 from both sides:} \\ 39x\text{ = 180 - 24} \\ 39x\text{ = 156} \end{gathered}`
`\begin{gathered} divide\text{ both sides by 39:} \\ (39x)/(39)\text{ = }(156)/(39) \\ \\ x\text{ = 4} \end{gathered}`
`\begin{gathered} m∠2\text{ = 18\lparen4\rparen + 6} \\ \\ m∠2\text{ = 78\degree} \end{gathered}`