Question

I need help with A and B please and thank you.

Answer 1

Given:

`\begin{gathered} 3y+6\ge5x \\ y\leq3 \\ 4x\ge8 \end{gathered}`

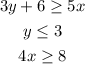
And we have that:

`\begin{gathered} 4x\ge8 \\ (4x)/(4)\ge(8)/(2) \\ x\ge4 \end{gathered}`

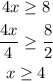
Therefore, both together imply that:

`10≤5x≤3y+6`

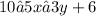
So we get that:

`10+3y≤5x+3y≤6y+6≤6\cdot3+6=18+6=24`

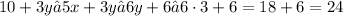
since we are given that y ≤ 3, so we also get:

`\begin{gathered} 10+3y≤6y+6 \\ 10+3y-6\leq6y+6-6 \\ 4+3y\leq6y \\ 4+3y-3y\leq6y-3y \\ 4\leq3y \end{gathered}`

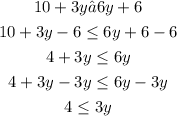
Now we have:

`4=10+4≤10+3y≤5x+3y≤24`

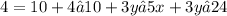
and then also the following:

a) The maximum of Q = 5x + 3y is 24, and the minimum of Q is 14.

Answer:

Maximum = 24

Minimum = 14

b) The new maximum would be the negative of the original minimum, and the new minimum would be the negative of the original maximum, therefore:

`14≤5x+3y≤24`

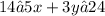
This is:

`\begin{gathered} 14≤5x+3y \\ and \\ 5x+3y≤24 \end{gathered}`

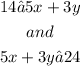
Then we can multiply both sides of both inequalities by -1, but we have to switch the direction of these inequalities:

`\begin{gathered} 14(-1)\leq5x(-1)+3y(-1) \\ -14\leq-5x-3y \end{gathered}`

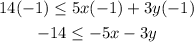
And

`\begin{gathered} 5x(-1)+3y(-1)\leq24(-1) \\ -5x-3y\leq-24 \end{gathered}`

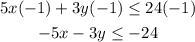
0r put in the correct order, from smallest to largest, we get:

`-24≤-5x-3y≤-14`

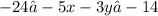
Answer:

Maximum = -14

Minimum = -24