Question

I need help finding the standard form of this equation

Answer 1

(x - 1)² + (y - 3)² = 36

Step-by-step explanation

The equation of a circle in standard form is,

`(x-h)^2+(y-k)^2=r^2`

Where (h, k) is the center of the circle and r is its radius.

As stated in the question, we have to complete the squares to find the equation of this circle in standard form. This means that we have to find a way to write the terms that contain each variable - x and y, in the form of a perfect binomial squared,

`(a+b)^2=a^2+2ab+b^2`

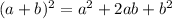
In the equation in general form, note that both x² and y² have the same coefficient, 3, and that all the other coefficients are multiples of 3. So, first, divide both sides of the given equation by 3,

`\begin{gathered} (3x^2)/(3)-(6x)/(3)+(3y^2)/(3)-(18y)/(3)-(78)/(3)=(0)/(3) \\ \\ x^2-2x+y^2-6y-26=0 \end{gathered}`

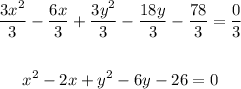
Now, let's take the terms that contain x: x² - 2x. As we can see, the first term of the binomial will be x, and the second term of the expanded form is -2x, so the second term of the binomial is,

`\begin{gathered} -2x=2ab \\ if\text{ }a=x \\ -2x=2xb\Rightarrow b=-1 \end{gathered}`

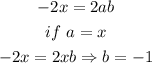
Hence, the binomial that contains x is (x - 1)², which expanded is,

`(x-1)^2=x^2-2x+1`

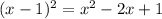
To replace the first two terms of the equation of the circle with this binomial we have to add and subtract 1, to keep the equality,

`\begin{gathered} (x^2-2x+1)-1+y^2-6y-26=0 \\ \\ (x-1)^2-1+y^2-6y-26=0 \end{gathered}`

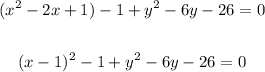
Similarly, for the terms that contain y: y² - 6y, we have that the first term of the binomial is y, and, if the second term of the expanded form is -6y, then the second term of the binomial is,

`\begin{gathered} 2ab=-6y \\ if\text{ }a=y \\ b=(-6y)/(2y)=-3 \end{gathered}`

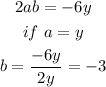
Hence, the binomial that contains y is (y - 3)², which expanded is,

`(y-3)^2=y^2-6y+9`

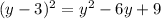
So, as we did with the binomial containing x, we have to add and subtract 9 to the equation of the circle to be able to replace the terms that contain y by this binomial squared,

`\begin{gathered} (x-1)^2-1+(y^2-6y+9)-9-26=0 \\ \\ (x-1)^2-1+(y-3)^2-9-26=0 \end{gathered}`

Finally, combine like terms,

`\begin{gathered} (x-1)^2+(y-3)^2+(-9-26-1)=0 \\ \\ (x-1)^2+(y-3)^2-36=0 \end{gathered}`

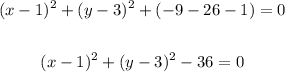
And add 36 to both sides,

`\begin{gathered} (x-1)^2+(y-3)^2-36+36=0+36 \\ \\ (x-1)^2+(y-3)^2=36 \end{gathered}`

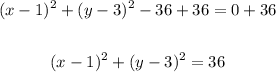
Hence, the standard form of the equation of this circle is (x - 1)² + (y - 3)² = 36, and the radius of the circle is 6, since 6² = 36.