In the quadratic equation
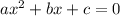
It has two roots, their sum = -b/a
their product is c/a
In our question the quadratic equation is
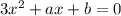
So the product of its root is b/3
One of the two roots is (1 - 2i), then the other root must be (1 + 2i)
Because its roots must be conjugate numbers
Let us find its product and equate it by b/3
(1 - 2i)(1 + 2i) = (1)(1) + (1)(2i) + (1)(-2i) + (-2i * 2i) = 1 +2i - 2i - 4i^2
2i + -2i = 0
i^2 = -1, then
(1 - 2i)(1 + 2i) = 1 - 4(-1) = 1 + 4 = 5
Equate b/3 by 5
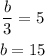
The value of b is 15