Question

Does 21.6 , 28.8, 36 form a right triangle

Answer 1

We will investigate the application of pythagorean theorem for right angle triangles.

A right angle triangle is denoted by one of its interior angle as 90 degrees. It has three side lengths denoted as follows:

`\begin{gathered} H\colon\text{ Hypotenuse ( longest )} \\ P\colon\text{ Perpendicular ( any of the two )} \\ B\colon\text{ Base ( last remaining )} \end{gathered}`

The pythagorean theorem relates the longest length of a right angle triangle ( H ) - hypotenuse with the other two side lengths of a right angle triangle by the following expression:

`H^2=P^2+B^2`

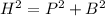
Lets say we have three side lengths of a triangle given as follows:

`21.6\text{ , 28.8 , 36}`

For the triangle with above denoted side lengths to be classified as a " right angle triangle" then it needs to conform to the pythagorean theorem states above. We will check whether the side lengths follows the pythagorean theorem or not.

`H\text{ = 36 ( largest ) , P = 21.6 , B = 28.8}`

Using pythagorean theorem:

`\begin{gathered} 36^2=21.6^2+28.8^2 \\ 1296\text{ = 466.56 + 829.44} \\ 1296\text{ = 1296} \end{gathered}`

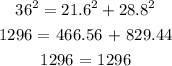
Since the right hand side equals the left hand side the pythagorean theorem is validated. This also means that the given triangle is a right angled triangle! Hence,

`\text{YES}`