Given:
There are given that triangle ABC.
Where,
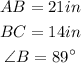
Step-by-step explanation:
According to the question:
We need to find angle C.
Then,
To find the angle C, first we need to find the value side AC.
So,
To find the side AC, we will use cosine function:
So,
let AC represents b.
So,
from the fomula of cosine:

Then,
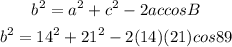
Then,
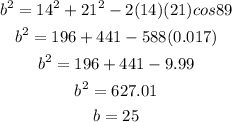
Now,
We need to use sine rule to find the angle C:
So,
From the sine rule:
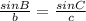
So,
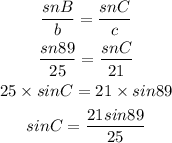
Then,
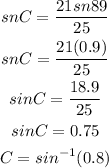
Then,
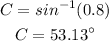
Final answer:
Hence, the value of measure angle C is shown below:
![C=53.13^{\operatorname{\circ}}]()